CPT Q. 071: What about the hydroplate model that assumes no pre-Flood ocean lithosphere existed?
Q. 71. Walt Brown’s hydroplate model assumes a pre-Flood earth that had no ocean lithosphere. In that model all of today’s ocean floor became exposed for the first time during the Flood. What about Flood models which assume that no ocean lithosphere as such was present in the pre-Flood world?
Response: As you point out, Walt Brown posits that the basic structure of the outer layers of the earth prior to the Flood was essentially uniform, with what would correspond to modern oceanic lithosphere everywhere covered with a layer of granitic crust some ten miles in thickness, with a 0.75 mile thick layer of liquid water sandwiched in between. One glaring problem with this idea is the lack of any conceivable mechanism for transforming that distribution of continental crust into the present one. In his book Brown pretends that issue does not exist and therefore provides no explicit answer for it.
It is noteworthy that through the 5th edition of In the Beginning, published in 1989, the thickness Brown assumed for the pre-Flood granitic crust was 10 km instead of 10 miles (16 km). In 1992 the Twin Cities Creation Science Association undertook what they called the Flood Model Project to compare hydroplate theory and catastrophic plate tectonics through a moderated back and forth written exchange between the two authors. In my initial critique of hydroplate theory, I pointed out that a critical requirement for the viability of the theory was an explanation of how the initial distribution of granitic crust, of a layer 10 km in thickness covering the entire earth, could be transformed into a layer roughly three times thicker covering only about a third of the earth’s surface. Somehow the initial layer would need to end up being stacked three layers high, like a stack of pancakes, where the continents exist today.
This is extremely difficult to do from a mechanical standpoint. For one thing, the layer of water hydroplate theory invokes to lubricate motion between the initial crustal layer and the underlying rock would not be available to lubricate the motion of one layer of crust onto another. Moreover, the forces and energy required to perform such a feat were far from having any obvious source. Especially problematic, I pointed out in the review, was how today’s Eurasian continent had its thickness increased by a factor of three during the Flood. Presumably, most of the crustal material responsible for such thickening had to have been derived for the area of today’s Pacific basin. This suggests that some of the crust had to move by some 10,000 km, or about a quarter of the way around the earth. There was no discussion of this problematic issue whatever in that edition of In the Beginning. I insisted that if hydroplate theory was to be taken seriously, this very glaring difficulty needed a coherent and satisfying explanation. At that point Brown withdrew from the review process.
Apparently Brown understood that this difficulty I identified during the review process was sufficiently important to prompt him to modify his theory. In subsequent editions of In the Beginning, he increased the thickness of the crustal layer from 10 km to 10 miles. Astonishingly, he removed some 30% of the earth’s surface corresponding to the present Pacific basin from the hydroplate process of rapid horizontal sliding. Instead, he simply had this crustal material sink a few kilometers into the earth. The increase in the initial crustal thickness, from 10 km to 10 miles, presumably was to compensate for the volume of crust which, in this revised framework, sinks beneath the Pacific basin. However, just as the earlier editions had given no hint that there was any problem in accounting for how the initial distribution of crust might be transformed into the one represented by the earth’s continents today, neither do the later editions. Certainly no serious discussion of this critically important aspect of hydroplate theory is to be found in the current edition.
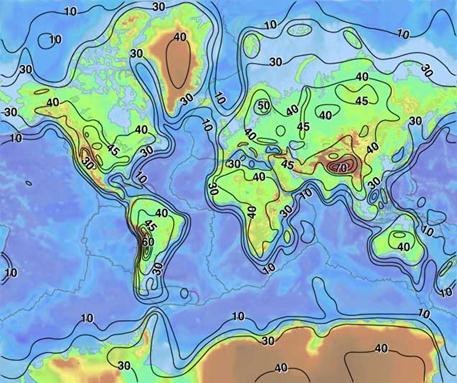
It is straightforward to evaluate Brown’s claim that a layer of buoyant granitic crust currently lies beneath the Pacific basin. The contrast between seismic velocities in granitic crust with those of mantle rock is extreme. Continental crust is readily distinguished from mantle rock by modern seismic techniques. The USGS crustal thickness map, displayed below, is based on such seismic data, primarily surface waves from earthquakes known as Love waves. Note the unmistakable absence of any thick layer of granitic crust in the Pacific basin.

Brown does, however, offer a mechanism to increase crustal thickness, one he compares to a train wreck. The process explained on pages 124 and 125 of In the Beginning involves first the displacement and acceleration of hydroplates away from the rising Mid-Atlantic Ridge, followed by an abrupt deceleration caused by the depletion of the water lubrication beneath each plate and/or by its encounter with a rigid obstacle. “As each massive hydroplate decelerated, it experienced a gigantic compression event—buckling, crushing, and thickening each plate.” On page 124 the analogy is made with the collision of a high-speed train with something rigid: “The long train of boxcars would suddenly decelerate, crush, and ‘jackknife’.” The basic mechanism, then, that Brown offers for thickening the crust is compressive shortening of the existing crust.
In regard to the details, the discussion is vague and qualitative. The only energy source Brown invokes is the kinetic energy of the moving crustal plate. From the words, “To illustrate this extreme compression, imagine yourself in a car travelling 45 miles per hour,” one infers a peak plate speed of 45 mph, or 20 m/s. Just how much mechanical work can this much kinetic energy accomplish? Very little. Specific kinetic energy, in terms of energy per unit mass, is given by 0.5v2, where v is the speed. For a speed of 20 m/s, the specific kinetic energy is [[0.5 \times 20^2 = 200 \text{ J/kg}]]. This is energy resident in every parcel of rock in the moving plate.
How much energy is needed to deform the layer so as to double its thickness? Let us utilize what is known as Byerlee’s law to estimate the stresses involved. This law is a formula Byerlee obtained from experimental investigation that gives the stress conditions in the earth’s crust at which fracturing along a geological fault takes place (for slightly more detail, see Wikipedia’s article on “Byerlee’s law”). Byerlee found that in the upper crust, the fracture criterion can be simplified to [[τ = 0.85σ_n]] for normal stresses up to 200 MPa and [[τ = 50 + 0.6σ_n]] for normal stresses higher than 200 MPa, where τ is the failure stress and [[σ_n]] is the normal stress, with both measured in MPa. A hydrostatic pressure of 200 MPa corresponds to a depth of 7.4 km when the crustal density is 2700 kg/m^3. In our case we want to estimate the level of shear stress required in compressing a layer of granitic crust originally 16 km thick to a final thickness of 32 km. Since we are mostly in the hydrostatic pressure range greater than 200 MPa (in other words at depths exceeding 7.4 km), for simplicity let’s apply the formula [[τ = 50 + 0.6σ_n]] everywhere.
A useful approach to applying Byerlee’s law is to average the normal stress [[σ_n]] (which we take to be equal to hydrostatic pressure) both in space and time. In the initial layer, [[σ_n]] at mid-depth in the layer, given by ρgd, where ρ is crustal density ([[2700 kg/m^3]]), g is gravitational acceleration ([[10 m/s^2]]), and d is depth (8000 m), is 216 MPa. When the layer is compressed to twice its original thickness, [[σ_n]] at mid-depth is twice as large, or 432 MPa. Averaged over time, the mean value of normal stress [[σ_n]] in the layer is 324 MPa. The mean shear stress τ, given by [[τ = 50 + 0.6σ_n]], then is 244 MPa. To convert this value of mean shear stress, which corresponds to work per unit volume done in compressing the layer, we simply divide by density to obtain ([[244 \times 10^6 J/m^3)/(2700 kg/m^3) = 90400 J/kg]]. This is some 450 times more energy that the kinetic energy available in a slab moving at 45 mph or 20 m/s, which we found above to be only 200 J/kg. This is not at all surprising, since deforming rock on this scale involves large forces and huge amounts of mechanical work. Thus, hydroplate theory seems to offer no obvious source of energy capable of compressing the original layer of granitic crust to twice its original thickness. This, of course, is a huge issue.
Yet there is an equally if not more serious issue, a geometrical one. Since in Brown’s scenario, the hydroplates, comprised of granitic rock, are sliding perpendicularly away from what today we refer to as an oceanic ridge, the volume of rock available for thickening the continent is restricted to the area between the ridge and the final trailing edge location of the thickened continent, multiplied by the original crustal thickness. Even a cursory glimpse of a map reveals the huge mismatches that result in this sort of crustal thickening scenario. So, not only is there no conceivable way from a geometrical standpoint to double crustal thickness in the present continents by moving crustal material in a perpendicular direction from a nearby mid-ocean ridge, but there is also no obvious energy source to allow it to happen.
What about actual physical observational evidence for compressional thickening of the continental platforms? Brown gives the impression that a ‘train wreck’ style of horizontal compression, which he argues must be responsible for the folding of the Canadian Rockies as shown in Figure 48 on page 112 of ITB, must also be responsible for increasing crustal thickness in the continent interiors—from 16 km to approximately 40 km—in regions where there are no mountain belts. Brown uses two exposures of the continental basement rocks, one in Black Canyon near Gunnison, Colorado, and the other from the inner gorge of the Grand Canyon in Arizona, shown in Figures 64 and 65 on pages 126 and 127 in &ITB*, to justify his contention that the deformations evident in these rocks occurred during the Flood.
But there is a major problem with this interpretation—even within the hydroplate framework. In hydroplate theory, as outlined on pages 120-127, the ‘Flood Phase’ occurs before the ‘Continental-Drift Phase’. The fossil-bearing sediments are formed during the ‘Flood Phase’, while the abrupt hydroplate deceleration and crustal thickening occurs at the end of the ‘Continental-Drift Phase’. As is so apparent in the Grand Canyon itself, and evident in Figure 65, the horizontally-bedded, fossil-bearing sedimentary layers that lie immediately above the crystalline rocks of the inner gorge have not experienced any dramatic horizontal shortening or chaotic deformation. If the crystalline basement rocks were compressed horizontally by a factor of at least two, in a ‘train wreck’ manner after the sedimentary rocks were in place, surely one would be able also to find evidence in these overlying sedimentary rocks of this same chaotic deformational process. But such chaotic deformation of sediments is systematically absent in all the continental platform regions of the world. Another important indicator that the hydroplate picture is not valid involves the radioisotope dates from these continental basement rocks which, interpreted in a relative sense, show that the rocks themselves, including the younger magmas which have intruded them, were in place before the Flood and must have been formed as part of God’s creation of the original earth.
In short, Brown’s hypothesis for the structure of the uppermost 100 km of the pre-Flood earth is not a viable hypothesis. There is no conceivable way to reconfigure a uniform layer of granitic crustal rock 10 km thick (using Brown’s original number) covering the entire earth into its present distribution in the continental cratons. The reality is that continental shield and platform areas display little indication of any significant internal deformation during that interval of geological history associated with the Flood.
Philip Budd’s model for the Flood, which like Brown’s has no pre-Flood lithosphere, takes a much different approach. It posits that the granite of the present continents formed deep within the mantle and during the antediluvian era rose as ‘hypomagma’ to the earth’s surface, ponding beneath a thin primordial crust beneath regions approximated by the present continents. According to the Budd hypothesis, the ‘collapse’ of and loss of ‘volatiles’ from this ‘vuggy’ hypomagma layer continued progressively ‘during the post-Flood millennium’. Accordingly, the continental crust and lithosphere as we know it today did not exist until at least a millennium after the Flood. Even more astonishing to me, Budd’s hypothesis is that essentially the entire fossil record, along with all the accompanying geologic and tectonic change recorded in that portion of the rock record, belongs to the post-Flood era, largely during the millennium after the main Flood cataclysm.
I consider such a scenario to be so strange and in conflict with so many readily available observations that I am at a loss to know where to begin in an evaluation. Certainly this scenario does not square with what seismology tells us about the state and structure of the mantle today, because if so much of it was molten in the recent past, presumably much of it ought to be today also, but it is not. It does not square with the structure of the modern seafloor, with its spreading ridges, its fracture zones, its trenches, its overall topography, its heat flow pattern, and its sediment distribution. It does not square with what is known about the crystalline basement of the continents, including its mineralogy and its distribution of radioisotope daughter products, including helium retaining zircons. In my assessment, Budd does not provide convincing justification for the pre-Flood state of the earth he is proposing.